|
No.521 |
Original Problems, Julia’s Fairies – 2014 (I): January – April →Previous ; →Next ; →List 2014(I) Please send your original fairy problems to: julia@juliasfairies.com |
No.521 by Chris Feather – An exotic four-men with neutral AUW and three fairy conditions
Definitions:
Anti-Kings: A king is in check if he is not attacked. Mate occurs when a king is not attacked and his side has no move which exposes him to attack. (Of course kings may not be captured.)
PWC: When a capture is made, the captured unit (except a King) is replaced on the square the capturing unit just leaves. A Pawn is immovable on its 1st rank.
Mars Circe: Non-capturing moves are normal, but to capture a unit is transported to its Circe rebirth square, which must be vacant, before proceeding to move towards the captured unit, all as one move.
An additional explanation by CJF: “Mars Circe works as follows: Captures occur only as if the capturing piece is starting from its game array square (determined as usual in Circe). Thus for example a black king can capture only by arriving on d8/d7/e7/f7/f8, as if from e8. In Mars Circe, captured pieces usually disappear as in normal chess, but in combination with PWC they reappear on the square just vacated by the capturing piece (NOT its game array square, unless of course the capturer actually plays from that square).“
|
No.521 Chris Feather
England
original-27.03.2014
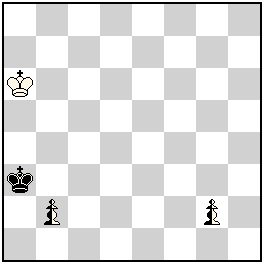 h#2 2 solutions (1+1+2) h#2 2 solutions (1+1+2)PWC Anti-Kings Mars Circe Hints: (click to show/hide)
Solutions: (click to show/hide)
|



There is a little typo in the explanation of the first solution:
the attack must be restored by creating a nB, which attacks the wK from f1 –> The nB attacks the wK from c8
This may well be the first problem composed by Chris with 3 fairy conditions. Despite the 3 conditions, this very neat Tanagra problem featuring AUW remains approachable by solvers – and all the more approachable if you are a ‘Fairings’ reader.
The explanation of the second solution is not clear to me. It’s wrong if my understanding of neutral pieces Mars circe is correct (of course, it might be vice versa):
-Neutral piece attacks the black pieces as from the rebirth square of its white component and the white pieces as from the rebirth square of its black component?-
If I’m wrong, what would be correct?
Does nSb1 attack wKa3? If not, why would nRa1 attack bKa3 as explained:
-“The nR goes to a1 (attacking the bK) so as to allow the nS-promotion without self-check, since the nS attacks only from g8”-
Also:
-“The bK cannot reach any of the squares attacked by this R (from h1) nor by the nS (from b8)…”-
Isn’t g1 relevant for nSa1’s attack on bK?
This combination of conditions takes a lot of effort to understand, but it is worth it!
Because of Mars Circe, the nPb2 attacks both Kings, because with Black to move the nP would go back to b7 before making a capture (on a6). Therefore neither King is in check, because of AntiKings!
(i) After 1…nPb3 both Kings are still attacked, because with White to move the nP would go back to b2 before making a capture (on a3). Once the nPb3 is captured, the attack on both Kings is removed. However, promotion to nBd1 ensures that the wK is attacked from c8 (not f1, as given by the author!). A nRd1 would also attack the wK (from a8), but Black could just move the nRd1 to a dark square, after which it would attack the bK from a1. Note that no nQ attack of the bK is possible, because the nBd1 occupies its rebirth square (which it must move to before making any capture). Also the bK cannot reach any square attacked by the nB from f1.
(ii) 1.nPg1=nR gives a second attack on the bK (from a1), allowing Black to move the nP without giving self-check. 2.nPb1=nS+ checks the wK, which is no longer attacked by the nP. However 2…nRxb1[nSa1] moves the nS onto a dark square, so the nS now attacks the wK from b8. The bK is no longer attacked, and it cannot reach any squares attacked by the nR from h1 or the nS from g1 (not b8 as given by the author!). Note that even if the nR moves to a dark square it does not attack the bK, because the nSa1 occupies the nR’s rebirth square.
I like the different promotions on g1 and the attacks on the wKa6 from nS (on b8) and bB (on c8).
And nSa1-b3/c2 would not attack bK because b1 is occupied.
I have asked: Why nPb2-b1=nS would be a selfcheck to bKa3?
Does not nSb1 attack a3?
Nikola, you are right about the explanation of the second solution. The purpose of the move 1…nRa1 is not to allow 2.nPb2-b1=nS without selfcheck to bKa3. There would be no selfcheck, since nSb1 attacks bKa3.
The purpose of 1…nRa1 is simply to reach a1, so that the captured nS occupies a1 after PWC-rebirth. This is the only way to ensure that Black cannot attack his King with the nR by moving it to a dark square.
Example: 1….nRc1? 2.nPb2-b1=nS nRxb1[nSc1]+ 3.nRb2+! and nRb2 attacks bKa3 from a1.
Thanks Eric!!!
So there is actually a mutual obstruction of Rb1 and Sa1 in the second solution! (Of course, the obstruction cannot be mutual in the first solution.)
However the idea is the same in the first solution: the nQ goes to d1 and captures nPb3 so that the reborn neutral Pawn occupies d1.
One example to make it clear:
1.nPg1=nQ nPb3 2.nQh1? nQxb3[nPh1=nB]+ 3.Ka4! and nQb3 attacks bK from d1.
Edit of the example I gave earlier for the 2nd solution: there is no check after 3.nRb2 because nSc1 attacks wKa6 from b8 🙂
A good clarification. Bd1 does obstruct Qb3, but Qb3 does not obstruct Bd1 (so the obstruction is not mutual).
I regard this not as a flaw in the first solution, but as a bonus of the second one. After all, the main theme is not obstructions but AUW.