|
No.219
|
Original Problems, Julia’s Fairies – 2013 (I): January – April →Previous ; →Next ; →List 2013(I) Please send your original fairy problems to: julia@juliasfairies.com |
No.219 – w1→ser-hs=18 by Paul Rãican – Interesting and long problem which has also a try! I’d like to comment also, that there’re two different approaches to show the number of moves in stipulation of this problem. Publishing Paul’s problem I’ve used the one introduced in the article Help-Self Problems with Black Series by IGM Petko A.Petkov. However author’s original number of moves in stipulation was 17. I’m showing the author’s version as well after editor’s version under the diagram. (JV)
Definitions:
The stipulation: w1→ser-hs=N means that White’s move is first, then Black makes a series of N-1 moves helping to realize as final an s=1.
Chameleon Chess: All pieces on the board which are displayed as orthodox Q, R, B, S, are Chameleons. A Pawn can promote only in Chameleon-pieces.
Chameleon: On completing a move, a Chameleon (from classical standard type) changes into another piece, in the sequence Q-S-B-R-Q… Promotion may be to a chameleon at any stage in the cycle.
Maximummer – Black must play the geometrically longest move or may choose from among longest moves of equal length, distances being measured from the center of each square. Diagonal and oblique distances are measured from the orthogonal coordinates by using Pythagora’s theorem (take the square root of the sum of the squares of the orthogonal distances). All other orthodox chess rules apply.
Sentinels: when a piece (Ks included but not pawns) moves, a pawn of the same color appears on the vacated square unless that square is on the first or eighth ranks or there are 8 pawns of that color on the board already.
|
No.219 Paul Rãican
Romania
original-01.01.2013
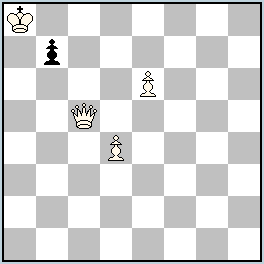 w1→ser-hs=18 (4+1)
Maximummer Sentinels Chameleon Chess Solution: (click to show/hide)
|



Yes, unfortunately, currently there are two ways of counting moves in serieshelpself-problems in use. The “old” way of counting (as Paul sent it) follows the pattern that is used for all series-help problems: a ser-h#n has n black moves in series followed by a #1, the same for ser-h=n – n black moves in series followed by a =1 etc. and so for ser-hs#n – it has n black moves in series followed by a s#1. You can find at least the following 15 problems before 2010 in PDB database for this kind of counting: P1189770, P1189970, P1191791, P1196281, P1205705, P1206150, P1206644, P1206944, P1207182, P1207183, P1207184, P1207374, P1207375, P1208066, P1210272.
Petko’s reasoning was that a ser-h#n has n-1 black moves and then a h#1, so a ser-hs#1 has n-1 black moves and then a S#1, thus introducing a new way of counting. (It is true that there was at least one occasion before his article that a similar counting was used – that is the No. 8 in his article.) Most of the serieshelpself problems after Petko’s article have been published with his new way of counting but not all. See my P1225008 and also P1227993 and P1228654.
Personally, I am for the “old” counting and do not see any serious reason why this should have been changed. Until there is a common “rule” that is accepted by all problemists, I will stick in my publications to the “old” way. Please note also that this is how Popeye is programmed. In the above problem you need to enter (after the first white move done) “ser-hs=17” if you just want to get the intended solution as Popeye was programmed according the “old” way of counting. The output of Popeye then shows the solution as in the “editor’s version” but that is just a computer program output and does not make it an 18-mover. Really, it does not make much sense to have the only white move listed in the 17th move and then the black response as 18th move as this is not the way how a s#1 or a hs#1 is really noted…
I just noted Seetharman’s comment to Petko’s article: “About No.8 Mr.Petkov expressed surprised that Popeye solved it as Ser.hs#12. I believe the Popeye team followed the logic that the solution required one to find the series of 12 help-moves, after which white forces mate. This reasoning seems to be fine to me.” For me too! Please look also at the solution of No. 13 in that article – obviously it was also published the “old” way…
In a self-mate/stalemate problem I would expect
n. white-move black-move #/=
not
n-1. … white-move n. black-move #/=
So, IMHO the editors version shoul at least end as
17. Rh2 18. Ka7 Qxc7
The answer is probably very easy, but please explain me the difference between ser-s#n and ser-hs#n. Sorry, I do not see it from first glance.
Please, ignore previous post.
By the article: traditional ser-s#N is the series of N-1 White moves and on the Nth move White forces selfmate in 1 move. While ser-hs#N is the series of N-1 Black moves.
But honestly, it doesn’t explain to me the fact why in ser-hs#N the last white half-move is added to (N-1)th move, but the last, Nth move, is just a half-move by black. This way it is shown in Petko’s article…
Nikola’s version seems logical, and I can’t understand why Petko recommends to write the solution differently.
“Traditional” ser-hs#N had a series of N black moves followed by a s#1 as the examples in PDB show. Obviously you can change a “tradition” if there are good reasons for that but I do not see those.
Petko wrote the solution as it is written in Popeye, but ignored the fact that Popeye just adds the N+1 move as the mating move as it was programmed like that.
I think the problem comes from the fact that ser-hs is not a “classical” series problem (series played by the same side + one terminal move).
But there is a way to transform it into such a classical series problem, by changing the goal, for example: ser-hs# = ser-h with goal “black must checkmate (B#)”.
The counting number is now unambiguous in this new setting, ser-hB#n means n black moves followed by a white move such that, in the resulting position, black has no other option than checkmating.