 Award of Julia’s Fairies 2013-III informal tournament
Award of Julia’s Fairies 2013-III informal tournament
(September – December’ 2013)
Judge: Eric Huber
107 problems by 42 authors from 15 countries took part in this fairy informal tournament. Moreover, the authors also came up with 10 versions, of which one was even a second version (456.2).
The number of problems (107) was so high that it suggested a splitting of the award in at least two sections. There are many possibilities when splitting a fairy award, for instance: according to the number of units, according to the presence/absence of fairy pieces or of fairy conditions, according to the type of stipulations (help, helpself, direct, series) and so on. After some hesitations, I decided to split it according to the number of pieces (54 miniatures vs. 53 non-miniatures).
In this judgment I tried to reward the density of fairy elements, the lack of formal defects as well as the originality of the ideas and the authors’ inventivity. The level was quite high and working on this award was a very satisfactory task.
One author’s output was especially notable, both in originality and quantity. His problems represent 30% of the published problems and 40% of the miniatures. Therefore it should not come as a surprise if you often encounter his name in the award and his identity will transpire very soon.
General remarks
Julia’s Fairies is a very special place to have one’s problem published, because comments about the published works are entered on a daily basis. This reactivity gives birth to lively, sometimes contradictious discussions and this explains how some issues related to fairy definitions were discussed on Julia’s Fairies:
- In problems 410 and 411 the stipulation Beidmatt was explained, as well as its difference with Gegenmatt and other stipulations – a commenter even provided a scan of Jörg Kuhlmann’s article in feenschach 1981.
- In 445 the definition of Take&Make Chess was clarified by its creator and the need for a new variant of this fairy condition was apparent.
- In 451 the exact definition of the Pressburger King, with all its implications, was also clarified in comments.
- Finally in 457 the problem of the legality of the setting (King in a corner in GridChess) was raised and closed.
A special case was problem 459. It did not receive any comment, because no one understood the solution. It would have deserved at least a question, because the white Poseidon is not mate after Black’s last move. Obviously the solution is the result of a bug in Popeye 4.63, which is regrettable. Later versions of the software no longer find this solution.
I propose the following ranking for the miniature and non-miniature sections.
Miniature Section
Two stimulating problems almost made it into the award:
- 386 (Daniel Novomesky) presents 6 solutions but not actual echo mates, although there are some similarities between pairs of mates. Perhaps the most interesting idea in the bunch was shown in the last solution, where the mate is given by the white King (the wK moves away from a field where it could serve as hurdle between a black Radial Leaper and the black King threatened by a white Radial Leaper). This idea would deserve to be extensively explored.
- In 412 (Peter Harris), the promotions of the two white Pawns are changed, but the black Rook also changes its function, making the solutions less unitary. Field e8 is potentially guarded by wKe2 in first solution, in the eventuality of a capture of wRa8 by bKf8, while e8 is guarded directly by wBc6 in the second solution. Too bad that the white King is not transmuted in the second solution. It remains an interesting and stimulating Tanagra.
|
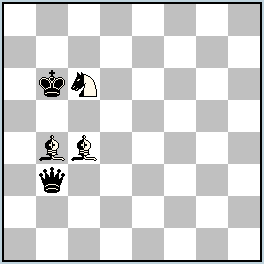
No.411 Peter Harris
South Africa
Julia’sFairies – 2013/III
5th Commendation
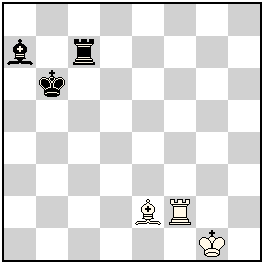 h##3 b) bKb6→a2 (3 + 3)
Transmuted Kings |
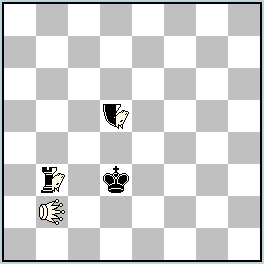
No.378 Paul Rãican
Romania
Julia’sFairies – 2013/III
4th Commendation
 1w→ser-hs=15 (1+1)
Chameleon Chess Maximummer; Sentinels |
|
a) 1.Rc7-c1+ Kg1-g5 2.Rc1-c4 Rf2-f7 3.Rc4-g4 + Kg5-b5## b) 1.Ba7-b8 Rf2-f1 2.Rc7-h7 Rf1-c1 3.Bb8-a7 + Be2-c4## |
Try: 1.Ke5-d5[+wPe5]? then 1.b7-b5 2.b5-b4 3.b4-b3 4.b3-b2 5.b2-b1=Q 6.Qb1-h7=S 7.Sh7-f8=B[+bPh7] 8.Bf8-a3=R 9.Ra3-h3=Q[+bPa3] 10.Qh3-c8=S[+bPh3] 11.Sc8-d6=B 12.Bd6-b8=R[+bPd6] 13.Rb8-b1=Q 14.Qb1-g6=S 15.Sg6-e7=B[+bPg6] 16.Be7-h4=R[+bPe7] & 1.e5-e6 Rh4-a4=Q[+bPh4] = too long. Solution: 1.Ke5-f6[+wPe5]! then 1.b7-b5 2.b5-b4 3.b4-b3 4.b3-b2 5.b2-b1=S 6.Sb1-c3=B 7.Bc3*e5=R[+bPc3] 8.Re5-a5=Q[+bPe5] 9.Qa5-d8=S[+bPa5] 10.Sd8-f7=B 11.Bf7-a2=R[+bPf7] 12.Ra2-h2=Q[+bPa2] 13.Qh2-b2=S[+bPh2] 14.Sb2-d3=B[+bPb2] 15.Bd3-h7=R[+bPd3] & 1.Kf6-g5[+wPf6] Rh7-h3=Q= |
5th Commendation 411 (Peter Harris)
Peter Harris was the composer whose prodigious output I referred to in the introduction. In Julia’s Fairies he proposed two problems featuring the stipulation ##, i.e. Beidmatt. This one is more homogeneous than 410 strategically speaking: in each solution, both Kings end up in similar mate positions. Original and pleasant to solve.
4th Commendation 378 (Paul Răican)
The try, with a stalemate that is distinct from the solution, fails because it is one move too long. Both lines of this Baby problem feature very specific play, which is to be expected with these fairy conditions. The problem would have been ranked higher if it had shown two equal length solutions.
|
No.379 Václav Kotěšovec
Czech Republic
Julia’sFairies – 2013/III
Dedicated to Juraj Lörinc
3rd Commendation
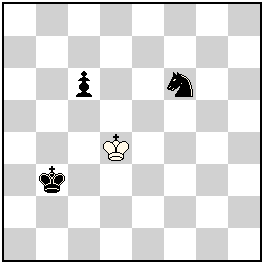 hs#4,5 3 solutions (1+3)
KoKo |
No.454 Adrian Storisteanu & Cornel Pacurar
Canada
Julia’sFairies – 2013/III
Plus c’est la même chose, plus ça change…
2nd Commendation
 h#2,5 2 solutions (0+3+2n)
Anti-Super Circe; PWC (no white King) |
|
I. 1… c5 2.Kc3 Sd5+ 3.Kb2 Ka3 4.Kb3 c4+ 5.Ka4 Sc3# II. 1… Se4 2.Kc4 c5+ 3.Kb5 Ka4+ 4.Kb6 Sd6 5.Ka5 Sc4# III. 1… Kc3 2.Kc5 Kb4+ 3.Kb6 Ka5+ 4.Kb7 Sd7 5.Ka6 Sc5# |
I. 1…nBd5xc6[nBc6->a2][+nSd5] 2.Rd4xd5[bRd5->b1][+nSd4] nBa2xe6[nBe6->c2][+bBa2]+ 3.Kd3xd4[bKd4->a1][+nSd3] nBc2xd3[nBd3->c3][+nSc2]# II. 1…nBd5xc6[nBc6->g8][+nSd5] 2.Rd4xd5[bRd5->h7][+nSd4] nBg8xe6[nBe6->g6][+bBg8]+ 3.Kd3xd4[bKd4->h8][+nSd3] nBg6xd3[nBd3->f6][+nSg6]# |
3rd Commendation 379 (Vaclav Kotěšovec)
A Köko triple chameleon echo is a common production for Vaclav: he has composed more than 80 Köko chameleon echo problems, one of them even in Julia’s Fairies 2012 (problem 53 with Grasshoppers) and 7 of them were helpselfmates. This present production is dedicated to Juraj Lörinc because of Juraj’s 2012 problem (see Appendix A). However, it does not have the length or special characteristics of 380 (see 2nd Prize).
2nd Commendation 454 (Cornel Păcurar & Adrian Storisteanu)
The 100% Anti-Super-Circe specific solutions are finalized with echo mates. The solutions are rather symmetrical, which somewhat mars the general impression.
|
No.417 Peter Harris
South Africa
Julia’sFairies – 2013/III
1st Commendation
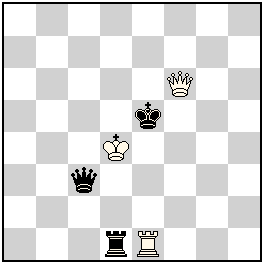 h=3 b) hs=3 (3+3)
Patrol; Sentinels Pion Advers |
No.394 Peter Harris
South Africa
Julia’sFairies – 2013/III
4th Honorable Mention
 h=2 (0+0+2n)
Sentinelles Pion Neutre Super-Circe |
|
a) 1.Ke5-e4[+wPe5] Re1-h1 2.Rd1-f1 Rh1-h3 3.Rf1-f5 Qf6xf5[+bPf6] = b) 1.Kd4-e4[+bPd4] + Qc3xe1[+wPc3] + 2.Ke4-d5[+bPe4] d4-d3 3.Kd5-d4[+bPd5] + Qe1xc3 = |
I. 1.nKa2-b2[+nPa2] + nKb2xa3 [+nPb1=nR][+nPb2] + 2.nRb1xb2 [+nPb3] nPa2xb3 [+nPa1=nB] = II. 1.nKa2xa3 [+nPb3][+nPa2] nKa3xb3 [+nPa1=nR][+nPa3] + 2.nRa1xa2 [+nPc8=nR] nRc8-c2 = |
1st Commendation 417 (Peter Harris)
The sentinels combined with Patrol Chess allow original ‘pinning’ effects: a black unit cannot leave a square, because a wP would appear that would cause a check to the bK by patrolling.
Uncommon both in conception and in twinning.
4th Honorable Mention 394 (Peter Harris)
Original combination of fairy pieces and conditions. The original stalemate positions do not need to be in echo to be interesting.
|
No.466 Peter Harris
South Africa
Julia’sFairies – 2013/III
Dedicated to commentator Seetharaman.
3rd Honorable Mention
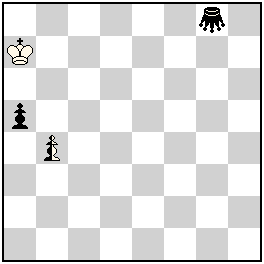 hs=3 b) neutral Ka7 (1+2+1n)
Maximummer; Super-Circe (no black King) |
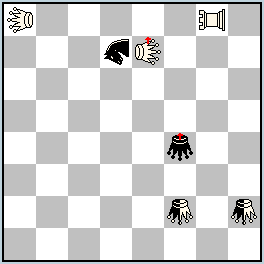
No.458 Ján Golha
Slovakia
Julia’sFairies – 2013/III
2nd Honorable Mention
 h#2 2 solutions (0+2+3n)
Take&Make; Anti-Take&Make (no white King) |
|
a) 1.nPb4×a5 [+bPh2] h2-h1=Q 2.nPa5-a6 Qh1-a8 + 3.Ka7×a8 [+bQb3] Gg8-a2 = b) 1.nPb4×a5 [+bPb7] b7-b5 2.nPa5×b6 ep.[+bPb1=bR] + Rb1×b6 [+nPa8=nG] 3.nKa7×a8[+nGg2] Gg8-g1 = |
I. 1.Qb3×c4-a6[+nBd5] nBb4-c3 2.Kb6xc6-a5[+nSb4] nSb4×d5-c4[+nBc6] # II. 1.Qb3×b4-a5[+nBd6] nSc6×a5-b5[+bQa7] 2.Kb6-a6 nSb5×d6-c5[+nBc7] # |
3rd Honorable Mention 466 (Peter Harris)
The uncommon and subtle twinning wKànK provides for completely different solutions. The wK prevents the solution from b) because 2.a5xb6 ep does not give check to the wK; the nK prevents the solution from a) because the longest move would not be 1…h1Q, but 1…nKb6/nKb8.
2nd Honorable Mention 458 (Jan Golha)
Two chameleon echo mates produced at the end of a play with good fairy specificity and variety, as noticed by commentators: a splendid Tanagra.
|
No.468 Peter Harris
South Africa
Julia’sFairies – 2013/III
Dedicated to Manfred Rittirsch.
1st Honorable Mention
 hs#2,5 Duplex (3+3)
Super-Circe Isardam |
No.382 Kostěj Šoulivý
Czech Republic
Julia’sFairies – 2013/III
3rd Prize
 h#2 4 solutions (1+1+2)
(bK is under check) Super-Circe Contra-Grasshopper b2 Nightrider d5; Empress b3 |
|
I. 1…Rd2-d3 2.Rd6-d5 + Qa8×d5 [+wRe2] + 3.Kd4-e3 + Kf3×e2 [+wRf3] # II. 1…Qh1-h4 2.Qa8-d5 + Rd6×d5 [+bQe5] 3.Kf3-e4 + Kd4×e5 [+bQd4] # |
I. 1.Kd3-c4 CGb2-b4 2.nNd5xb4 [+wCGf4] nEMb3xb4[+nNe4] # II. 1.Kd3-e2 nNd5-h3 2.nEMb3xh3 [+nNf2] nEMh3xf2[+nNc2] # III. 1.Kd3-c2 nNd5-f4 2.nNf4xb2 [+wCGf2] nEMb3xb2[+nNe2] # IV. 1.nNd5-c3 CGb2-b5 2.nEMb3xb5 [+wCGg3]nEMb5xc3 [+nNf3] # |
1st Honorable Mention 468 (Peter Harris)
The Qs and Rs reciprocally exchange their functions in the Duplex solutions. This is definitely an original concept and an original use of the combination (Super-Circe+Isardam) Peter Harris is a specialist of. The number of SuperCirce effects in the solutions is unfortunately reduced, but it would have been a miracle to have more with this material.
3rd Prize 382 (K. Šoulivý)
This pure product of the Bohemian school displays four chameleon echo mates. The play is unified play with two Super-Circe rebirths in the last two half-moves of each solutions.
That the black King is in check in the diagram position does not disturb me. However, the nN is used as a nS in two solutions and this is slightly bothering.
A technical note: replacing CG with Lion does not work due to cook 1.Ke2 nNf1 2.Kxf1[+nNc1] nEMxc1[+nNd2]#
|
No.380 Václav Kotěšovec
Czech Republic
Julia’sFairies – 2013/III
2nd Prize
 hs#9 3 solutions (1+3)
KoKo |
No.390 Mario Parrinello
Italy
Julia’sFairies – 2013/III
1st Prize
 hs#3 b) rCGe7↔NAd7 (3+2+2)
Royal Grasshopper f4 Royal ContraGrasshopper e7 LEO a8; PAO g8; NAO d7 Grasshoppers f2, h2 |
|
I. 1.Kc5 Kd6 2.Kd5 Qd4+ 3.Ke4 Qe3 4.Kd5 Ke5 5.Ke6 Kf6+ 6.Kf7 Kg7 7.Kg8 Kh8 8.Kg7 Th6+ 9.Kg8 Qg5# II. 1.Kb5 Qa6+ 2.Kc5 Kd5 3.Kd4 Ke4 4.Ke3 Kf3 5.Kf2 Te1+ 6.Kg3 Kg2 7.Kh2 Kh1 8.Kg2 Tf1+ 9.Kh2 Qe2# III. 1.Kb7 Tb1+ 2.Kc7 Tb8+ 3.Kd6 Qe5+ 4.Ke6 Qf5 5.Kd6 Kb7 6.Kc6+ Ka8 7.Kc7 Td8 8.Kb7 Tc8+ 9.Ka7 Qd7# |
a) 1.rCGc7 NAg1 2.PAf8 nGe5 3.LEf3 + rGd6 # b) 1.rCGf7 NAh1 2.LEb8 nGf5 3.PAg3 + rGf6 # |
2nd Prize 380 (Vaclav Kotěšovec)
Incredible triple echo in three different corners.
The long solutions and the far echoes make this problem a very peculiar instance of Köko echoes.
1st Prize 390 (Mario Parrinello)
The relative wealth of fairy units (6 types, belonging to 2 different families, the Chinese and the Grasshoppers) explains why approaching this problem is difficult. Once the solutions are understood and the strategic contents are assimilated, some commentators may wonder if this mix of different fairy units justifies the effort.
Nevertheless, thanks to the seamless diagonal-orthogonal correspondence between solutions and the whole geometric beauty of the work, it earns its place in the award.
Non-Miniature Section
As a preliminary remark, a note about 465 (S. K. Balasubramanian & K. Seetharaman).
The good unity in the orthogonal-diagonal royal battery creation was somewhat spoilt by some defects that prevented its inclusion in the award: in b), W1 makes a capture; in a) Vao and Leo are doubled on the diagonal a3-f8 and a wP guards a flight of the bK to a5. Moreover, a presentation with bQh1 would have been more economical. (White LEa8 VAd8 LEh8 Pb5 Pb4 Ka1 Bf1 – Black Pc7 Pd7 Pb6 Kb3 Pc2 Qh1 with same stipulation and twin).
|
No.418.1 Eugene Rosner
U.S.A.
Julia’sFairies – 2013/III
5th Commendation
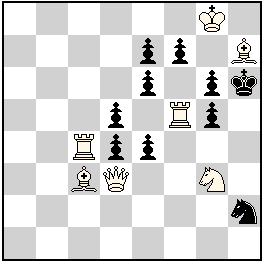 #2 (7+10) C-
Alsatian Circe |
No.405 Peter Harris
South Africa
Julia’sFairies – 2013/III
4th Commendation
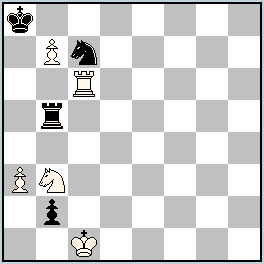 hs#3 b) wPa3→f4 (5+4)
Transmuted Kings Einstein Chess; Isardam |
|
1.Qd2? Sf3! 1.Rf6! threat (2.Rxg6(Pg7)#) 1…ef6(Ra1), 2…fg6(Rh1)? 1…ed(Qd1) 2.Qh5# (2.Sf5? ef5(Rh1)!) 1…dc3(Bc1) 2.Bxg5(Pg7)# (2…Kxg5(Bc1)+?) 1…dc4(Rh1) 2.Rxh2(Sb8)# |
a) 1.Rc6-b6=B Rb5-b4=B 2.Bb6xc7=R + Ka8-a5 3.a3xb4=S + Ka5xb3 # b) 1.b7-b8 Ka8-b7 2.Rc6xc7=Q + Kb7-d5 3.Qc7-e5=R + Rb5xb3=Q # |
5th Commendation 418.1 (Eugene Rosner)
The author shows an original use of Alsatian Circe in direct mate thanks to a specific motivation of play. The fairy density is quite appropriate as far as Alsatian Circe is concerned: all variations (moving bPs toward the West of the board) are motivated by the need to enable the capture fxg6 and all white mates are made possible in turn by the rebirth of a white unit.
Finally, as the author observed, the dual-avoidance in the first variation (1…exd3(Qd1) 2.Sf5+? exf5(Sb1)! because the capture is allowed by the Alsatian stipulation) is an integral part of the problem’s theme.
At this point, I cannot refrain from quoting Theodor Tauber’s 1982 Alsacian Circe problem from 1982 (Appendix B): shouldn’t there be more such problems intermingling retro and fairy motives?
4th Commendation 405 (Peter Harris)
Over the years Peter Harris has composed several problems with the combination of Einstein+Isardam. With Transmuted Kings it is an amazing mix of fairy conditions. In the troubling initial position, the Kings are not in check because in Einstein a capturing pawn changes into a S and both Kings are guarded by S, making the capture illegal according to Isardam.
Fairy conditions dictate move order and avoid duals (example in twin a) 1.Rc6-d6=B? makes impossible 1…Rb5-b4=B due to Isardam) and are all necessary to the solution and the final selfmates.
|
No.421 Georgy Evseev
Russia
Julia’sFairies – 2013/III
3rd Commendation
 hs#3 2 solutions (7+8)
Circe PAOs: g2, h1; VAO f3 |
No.401 Franz Pachl
Germany
Julia’sFairies – 2013/III
2nd Commendation
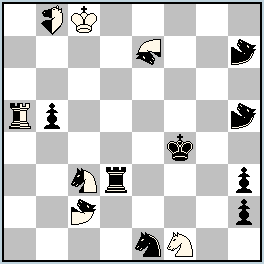 h#2 3 solutions (2+8+5)
Take&Make Rose b8; Camel c2 h7 h5; Zebra e7 |
|
I. 1.c7-c8=B Rd8xc8[+wBf1] + 2.Bf1-d3 Rc8-c6 3.Ba6-b7 + Ka8xb7[+wBf1] # II. 1.c7xd8=S[+bRh8] Rh8xd8[+wSg1] + 2.Sg1-h3 Rd8-b8 3.Sd7-b6 + Rb8xb6[+wSg1] # |
I. 1.CAe4 nSxb5-b4 2.Rd5 nSxd5-h5# II. 1.CAag4 nnZxb5-b4 2.Rd7 nZxd7-h7# III. 11.Sf3 nCAxb5-b4 2.Re3 nCAxe3-e1# |
3rd Commendation 421 (Georgy Evseev)
The original feature of this problem is the following: the promoted unit is captured and moves to free the rebirth square and provide an anticipatory block of a line/square for the next reborn unit. As a further fairy specificity: in the mating position, the white reborn unit cannot capture the black PAO (respectively VAO) because it would be reborn on the 1st rank on g1 (resp. f1) and would serve as a hurdle for PAOh1.
Unfortunately black play at B2/B3 is heterogeneous: the bR plays to selfblock in twin B, to interfere with bVAO in twin A. Another defect is that the scheme has static Chinese pieces – in fact only 4 units out of 15 happen to move during the two solutions.
2nd Commendation 401 (Franz Pachl)
The first black move is a self-block and by clever construction also a square vacation. The blocked flight was initially guarded by a neutral unit and the same neutral unit will arrive on the vacated square on the last move, after a Rose battery play and the unpinning and capture of bRd3.
The strategic complex would have been even more convincing if the created Rose battery had been set on different fields: the repetition of the capture of bPb5 on the 1st move is monotonous and the global fairy density is not too high.
A marginally more economic version getting rid of wSf1 would be –wSf1, –bPh2, bPh3àg3, +nPd2 (1+7+6).
|
No.442 Juraj Lörinc
Slovakia
Julia’sFairies – 2013/III
1st Commendation
 hs#4 (5+6)
b) Kd1→e1 ; c) Kd1→f1 LEO b5 ; PAOs: a5, d4 |
No.472 Petko A. Petkov
Bulgaria
Julia’sFairies – 2013/III
New Year Greetings!
7th Honorable Mention
 h=2 (3+2+4)
b) Ka5→b2; c) nPAf4→e2 Disparate VAO g6; PAO f4; NAO b5 |
|
a) 1.Sd2 PAe4 2.Sb3 PAe1 3.Ke4 f×g5 4.LEa4+ Ke2# b) 1.Sc3 PAf4 2.b×c5 PAf1 3.Kf4 f5 4.LEb4+ Kf2# c) 1.S×c5 PAg4 2.Rh5 PAg1 3.Kg4 g5 4.Sd3+ Kg2# |
a) 1.nNAb5-d6 nPc7-c8=nNA 2.nVAg6-d3 nNAd6-b2 = !! b) 1.nPAf4-a4 nPc7-c8=nPA 2.nVAg6-f5 nPAa4-a5 =!! c) 1.nVAg6-c2 nPc7-c8=nVA 2.nNAb5-f3 nVAc2-d1 =!! |
1st Commendation 442 (Juraj Lörinc)
The three royal antibattery mates are built in the same, rather mechanical way. The author has ensured that the solutions contain plenty of unity, but concomitantly the white play brings variety and inventivity to the problem. In my opinion, this variety does not detract. However, wRg5 is underused.
7th Honorable Mention 472 (Petko A. Petkov)
The presented cycle is undoubtedly original: cyclic double pins nPA/nVA (AB), nVA/nNA (BC), nNA/nPA (CA) combined with double half-moving Disparate-Py paralysis of both neutral pieces (nNAnNA, nPAnPA and nVAnVA) for Black. There are some similarities with Roméo Bédoni, 1st Commendation, Phénix 2004 (Appendix D): move order determined by promotion, pin stalemate and Disparate paralysis in the final position. But the author of 472 refined and developed the theme in 3D instead of 2D.
There is a slight imbalance in twins due to the imperfect exchange of functions during the solutions: the neutral Vao plays twice at B2 (in twins A and B), when the neutral Pao should have played once. The problem would be better without this defect, but removing it is technically difficult. In that sense, the version proposed by G.Evseev was a good starting point, despite having other defects in the author’s opinion.
|
No.447.1 Pierre Tritten
France
Julia’sFairies – 2013/III
6th Honorable Mention
 h#2 2 solutions (6+12)
Take & Make |
No.373 Julia Vysotska
Latvia
Julia’sFairies – 2013/III
5th Honorable Mention
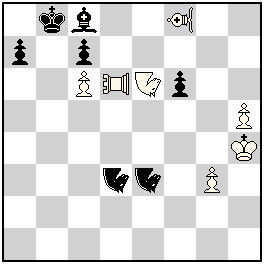 hs#3 2 solutions (7+7)
Take&Make PAO d6; VAO f8; Nightriders: d3,e3,e6 |
|
I. f×g6-b6 h×g3-e3 2.Q×c2-h7 R×h7-c2‡ II. f×g6-c6 h×g3-b3 2.Q×a7-h7 B×h7-Ba7‡ |
I. 1.Ne6-c2 Ne3xc2-a3 2.VAf8xa3-e5 Nd3-f4 3.PAd6-d8 + Nf4xd8-d6 # II. 1.Ne6-f4 Nd3xf4-g6 + 2.PAd6xg6-h8 Ne3-c4 3.VAf8-d6 + Nc4xd6-f8 # |
6th Honorable Mention 447.1 (Pierre Tritten)
This Take&Make problem presents in a compact way some typical fairy effects, of which the obstruction-and-removing that would require more moves in orthodox chess. All moves require captures for various motives. It must be noted that the key has an AntiZielElement, because it gives the black King a flight by annihilating the piece guarding it. The black Queen’s ‘invisible captures’ as per WCCT-10 terminology (case where square A = square B) are especially striking.
5th Honorable Mention 373 (Julia Vysotska)
As Juraj Lörinc commented on Julia’s Fairies, the basic idea of parrying double check by very precisely determined Take&Make capture could also be found in Petko Petkov, 4th Prize StrateGems 2011, Appendix C.
But the author justifiedly underlined that the main mechanism is different. The reciprocal play by Pao/Vao enables the building of reciprocal batteries Pao/Vao for White and the bNs exchange their functions (Zilahi, but also all Black moves). All this is achieved in a dynamic and pleasant play with many active sacrifices.
|
No.429 Petko A. Petkov
Bulgaria
Julia’sFairies – 2013/III
Dedicated to the memory of the victims of Riga tragedy!
4th Honorable Mention
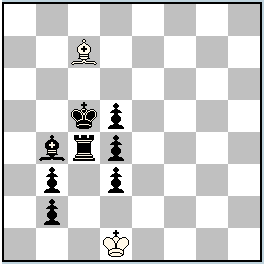 h#2 b) Bc7→d7 ( 2+8 )
Disparate Anti-Andernach |
No.460.1 Georgy Evseev
Russia
Julia’sFairies – 2013/III
3rd Honorable Mention
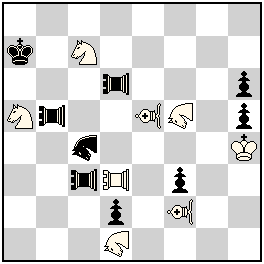 hs#3 2 solutions (8+9)
NAOs: d1, f5, c4 PAOs: b5, c3, d6 VAOs: e5, f2 |
|
a) 1.b2-b1=wB!! Bb1xd3 2.Rc4-c1=wR!! 2… Bd3-b5=bB #!! b) 1.b2-b1=wR!! Rb1xb3 2.Bb4-a3=wB!! 2…Rb3-b6=bB #!! |
I. 1.NAb3 PAa6 2.PAd4+ NAb6 3.NA×h6 PA×h6 # II. 1.PAd5 NAa8 2.NAd4+ PAdb6 3.PA×d2 NA×d2 # |
4th Honorable Mention 429 (Petko A. Petkov)
The Disparate condition, at least as it is implemented in Popeye, enables strange effects. For instance with Anti-Andernach, promotion is the only move that allows the same unit to move twice consecutively. And the unit that moves at the mating move is never the unit that gives the mating check, because it will be paralyzed at the next half-move. White must prepare beforehand the attack of the black King or, if Black collaborates nicely in Anti-Andernach, Black himself plays a paradoxical move that apparently puts his own King in check (by 2.Rc4-c1=wR and 2.Bb4-a3=wB). The author called this ‘creation of a Disparate battery’ and Julia named it a ‘deferred self-check’.
The author also underlines the blocking of squares by the white pieces which change their color to black, and this effect is also specific of the combination Disparate + Anti-Andernach.
On the whole, this work is a successful helpmate with high fairy density and originality.
As a technical side note: interestingly, the white King is not needed on the board. However, the wK cannot be put on e5 to spare some blocking pawns (d5-d4) because of the Disparate mate 1.Kd5#
3rd Honorable Mention 460.1 (Georgy Evseev)
The self-obstruction by the black units (Nao and Pao), a difficult theme, is well done. The problem is also a welcome deviation from the usual diagonal-orthogonal correspondence.
In the initial position, bRb5 and bPAc3 are pinned by the wNAd1: these technical units are hard to spare. The problem also fulfils the WCCT9 Theme thanks to the critical moves of wNAf5, resp. wPAd3 and of bNAc4, resp. bPAf6. These moves by the thematic white pieces enable the capture of a black pawn, which will provide the single possible black move on B3.
The version 460.1 eliminated a slight defect (the fact that wPA ‘tries’ were regrettably not echoed in the wNA variation) and another more serious defect (wSc7 was useless in one solution). The opening of the diagonal of wVAe5 by bPAd6 is not replicated by bNAc4 and Petko Petkov proposed a version to remedy that inconvenience. However, that feature is completely secondary to the strategic complex and whether the Bulgarian version or the Russian version is to be preferred is a matter of taste.
|
No.376 Petko A. Petkov
Bulgaria
Julia’sFairies – 2013/III
Dedicated to Julia Vysotska at her birthday 16.09.2013!
2nd Honorable Mention
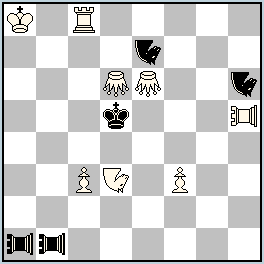 hs#2,5 2 solutions (8+5)
Take & Make Nightriders: d3, e7, h6 Grasshoppers: d6, e6 PAOs: a1, b1, h5 |
No.392.1 S.K.Balasubramanian
India
Julia’sFairies – 2013/III
Dedicated to Julia Vysotska
1st Honorable Mention
 hs#2,5 2 solutions (6+7)
Take & Make Locust h5 |
|
I. 1…Nd4 2.Gxd4(Gb3) Nh1 3.Nxh1(Nf5)+ Kxe6(Ka2)‡ II. 1…Nc6 2.Gxc6(Gb4) Nf7 3.Nxf7(Ng5)+ Kxd6(Ka3)‡ |
I. 1…Rf4-f3 2.Kb6xc7-c2 Lh5xh2-c1+ 3.Sd5xf3-c3+ Rf3xc3-d5 # II. 1…Bf6-d8 2.Kb6xc7-g7 Lh5xh2-h8+ 3.Sd5xf4-f6+ Bd8xf6-d5 # |
2nd Honorable Mention 376 (Petko A. Petkov)
These difficult solutions with anti-batteries K-Pao and N-Pao present 2 exchanges of functions Gd6/Ge6 and Ne7/Nh6 and two sacrifices by bNs in each solution. The uniqueness of the solutions is guaranteed by the need to guard by the wN the square vacated by the wG.
Construction is excellent and the solutions, as intended by the author, are difficult.
1st Honorable Mention 392.1 (S. K. Balasubramanian)
A Locust is taboo in Take&Make and cannot be captured, as the normal move of a Locust is a capture move and the ‘Make’ move can’t be a capture move. This aspect is not shown for the first time in Take&Make problems (see for instance the previous Petko Petkov, 4th Prize StrateGems 2011, Appendix C). Here, the Locust inflicts orthogonal and diagonal mates and collaborates smoothly with the remaining black unit.
With relatively few pieces on the board, the high fairy density is remarkable.
|
No.396 Peter Harris
South Africa
Julia’sFairies – 2013/III
Special Prize
 h=2,5 (6+9)
a) Anti-Circe Cheylan + Super-Circe b) Anti-Circe + Super-Circe |
No.377.1 Igor Kochulov
Russia
Julia’sFairies – 2013/III
5th Prize
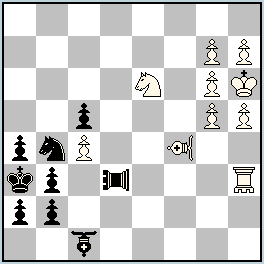 hs#3 Duplex (10+9)
Rookhopper h3; Bishophopper c1 PAO d3; VAO f4 |
|
a) 1…Kh1-h2 2.f2-f1=R Kh2xg2 [+bPe8][wKg2->e1]3.b3-b2 Ke1xf1 [+bRb1][wKf1->e1] = b) 1…Ra8xa1 [+bRa8][wRa1->a1] 2.Ra8xc8 [+wSa2][bRc8->a8] + Kh1xg2 [+bPb8][wKg2->e1] 3.b3xa2[+wSa6][bPa2->a7] Ke1xf2 [+bPe8][wKf2->e1] = |
1.VAe3 Pad2 2.VAf4+ Sd3 3.VAd6+ PAxd6# 1.PAe3 VAg3 2.PAd3+ Sf4 3.Pad6+ VAxd6# |
Special Prize 396 (Peter Harris)
Twinnings using the differences between Anti-Circe Cheylan and AntiCirce Calvet are usually a complex matter. A renowned specialist of this type of twinning is Klaus Wenda. Therefore some explanations may be useful for the reader in order to understand this complex problem.
The solution of a) fails in b) because Black could play 4.Ra1xa8 [+wRa1][bRa8->a8]. The solution of b) is illegal in a) because Black is not allowed to play 1…Ra8xa1 [+bRa8][wRa1->a1] in Anti-Circe Cheylan.
In the final position of twin a) the black pieces on a1-c1-d1-g1 are specifically pinned by the hypothetical promotion of wPg7 by capture of bKh8, which would be followed by the rebirth of the promoted pawn on a1 in case of Rook promotion, c1 for Bishop promotion, d1 for Queen promotion and g1 for Knight promotion. Besides, the capture of wPg7 by the bK or bQ are forbidden due to the occupation of their rebirth squares d8 and e8.
In the solution of b) a sarcophagus is built in the NW corner for the bRa8 and it is locked by bSa6. The fact that a different black Pawn blocks e8 in each solution is the cherry on the cake.
The excellent twinning (Anti-Circe Cheylan/Calvet) enables completely different solutions showing the rich possibilities of the rebirth on capture square. The second solution is especially spectacular with its 100% specific moves and 4 Super-Circe rebirths. The slight unbalance between solutions made me grant this problem a special award.
5th Prize 377.1 (Igor Kochulov)
In Duplex the thematic exchanges are not limited to one color. Here the pairs of units reciprocally exchanging their functions are bPAOd2/wVAOf4, bVAOc1/wPAOh3 and bSb4/wSe6. The orthogonal-diagonal change between the two solutions is an impressively pure geometrical achievement spiced up with varied pins and unpins.
The visual impression is somewhat marred by the piles of black Pawns and white Pawns but adding all these blocks could hardly be avoided. The originality of the conception raises this problem to this high reward.
|
No.400 Julia Vysotska
Latvia
Julia’sFairies – 2013/III
Dedicated to Petko A. Petkov!
4th Prize
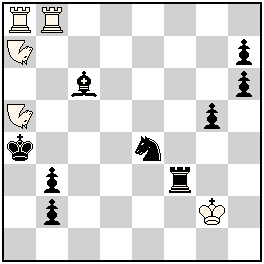 hs#3 2 solutions (5+9)
Take&Make Nightriders: Na5, Na7 |
No.370 Michal Dragoun & Ladislav Salai jr.
Czech Rep. / Slovakia
Julia’sFairies – 2013/III
3rd Prize
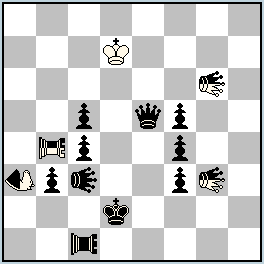 h#2 3 solutions (1+10+4n)
Anti-Circe Lions: c3, g3, g6 Rook-lions: c1, b4 Rose a3 |
|
I. 1.Na7-b5 Se4-g3 2.Kg2xg3-h5 Bc6xb5-d1 3.Na5-e3 + Rf3xe3-a5 # II. 1.Na5-b7 Rf3-f1 2.Kg2xf1-b1 Bc6xb7-f5 3.Na7-c3 + Se4xc3-a7 # |
I. 1.Qc7+ nLIxc7(nLIc8) 2.Kc2 Kxc8(Ke1) # II. 1.Qd6+ nROxd6(nROd8) 2.Kd3 Kxd8(Ke1) # III. 1.Qe4 nLIxe4(nLIe8) 2.Ke3 Kxe8(Ke1) # |
4th Prize 400 (Julia Vysotska)
Amateurs of battery play will be delighted by this problem. The white and black half-batteries both play and thus the two front pieces of each half-battery exchange their functions. The initial black half-battery transforms into two new black batteries created on different lines. In the same time, the active white King enables mates on different fields and lines.
This strategic complex loaded with battery play was a worthy dedication to GM Petko Petkov!
3rd Prize 370 (Michal Dragoun & Ladislav Salai jr.)
Readers interested in antibatteries in AntiCirce h#2 with orthodox pieces can have a look at the award of the 3rd round of the 2007 Liga Problemista, published in MatPlus 27.
Here the authors offer specific AntiCirce battery motivation for fairy units: the rebirth square is common for two fairy units that give check after the wK captures the fairy piece that occupied the rebirth square.
What results is a cyclic exchange of function between 3 neutral units as well as a cyclic Zilahi and an impressive aesthetic achievement.
|
No.445 Vlaicu Crișan
Romania
Julia’sFairies – 2013/III
2nd Prize
 hs#4 b) bPh4→e8 (11+9)
Take & Make type 1st rank |
No.443 N.Shankar Ram
India
Julia’sFairies – 2013/III
1st Prize
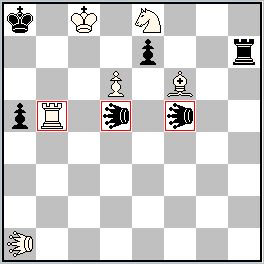 r#2 (6+6)
Elans: a1, d5, f5 Paralysing Pieces: b5, d5, f5 |
|
a) 1.Qc8 Rxg6-f7 2.Se6 Bxh5-f5 3.Bxc5-b3+ Kxb3-d1 b) 1.Qf1 Bxh5-h3 2.Sf3 Rxg6-f5 3.Rxd3-b2+ Kxb2-b6 |
Key 1.Kc7! (2.pRb7, Kxb7#) 1…Pxf6+ 2.d7, Rxd7# |
2nd Prize 445 (Vlaicu Crişan)
A heated discussion took place on Julia’s Fairies concerning the legality of this problem. The inventor of Take&Make condition personally confirmed that his definition explicitly excludes the possibility of pawn rebirth on 1st rank. The author, unable to find a convenient and economical setting without bPe8 in twin b), opted for creating a new condition named ‘Take&Make type 1st rank”. This is the condition that should appear under the diagram (definition can be found in this comment – in short, the difference with Take&Make is that pawns can move to the first rank of their own color where they are subsequently immobile.)
Now have a look at the complicated and eventful solutions that can be replayed here: 6 moves out of 8 are captures and pieces fly all over the place, especially both active Kings, in a weird ballet involving 4 pairs of units that reciprocally exchange their functions. During the solution, an indirect white royal battery and a direct black battery are created and many lines are opened and used for the complex strategy. This problem dwarfs previous achievements in Take&Make helpmates or helpselfmates and reminds of Vlaicu’s 1st Prize in the FIDE Olympic Tourney, 2012 (hs#3 Take&Make).
A real blockbuster, as it was called by Shankar Ram, but not devoid of subtlety.
1st Prize 443 (N. Shankar Ram)
Shankar Ram has tamed the Moose to show us a black correction of the 4th degree and the best problem of the tourney.
The great key offers two additional flights and exposes the white King to four different battery checks by Pickaninny moves. All this is achieved in Meredith form for the first time – although, truth be said, quaternary black correction has already been achieved in Meredith and without orthodox units, and black correction of a lesser degree has been achieved many times in combination with Pickaninny. The author himself composed a very nice reflex twomover with quaternary black correction – see appendix E for the pleasure of it).
It is probably interesting for the reader to detail the four cumulative weaknesses of the black Pawn’s moves, in the author’s words:
- 1..exf6 : opening of line h7-c7 (1st degree)
- 1..exd6: opening of line h7-c7/capture or paralysis of wPd6 (2nd degree)
- 1..e6: opening of line h7-c7/ capture or paralysis of wPd6/paralysis of wBf6 (3rd degree)
- 1..e5: opening of line h7-c7/ capture or paralysis of wPd6/ paralysis of wBf6/closing of the Moose line a1-f6-f7 (4th degree)
The author managed the trick by the astute disposition of the paralyzing units (black Moose and white Rook) and of the dual-avoiding white Moose.
Appendix
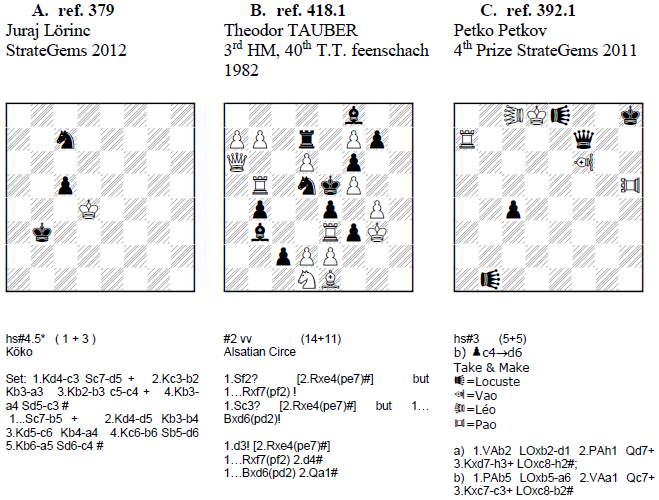
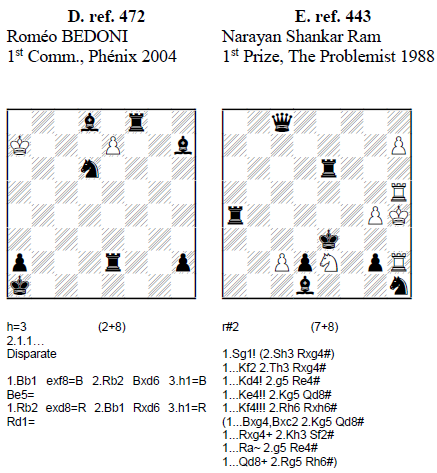
My congratulations to the awarded composers and my thanks to Julia, who asked me to act as a judge and who kindly put up with the delay in this judgment.

Eric Huber
Bucharest, June 30th 2015.


Nicely analysed award by Eric Huber. Very happy to see Shankar Ram in the prize list again, that too with a well deserved first prize. Congrats to all the other winners too!